《概率论与数理统计英文版总结.docx》由会员分享，可在线阅读，更多相关《概率论与数理统计英文版总结.docx（11页珍藏版）》请在第壹文秘上搜索。
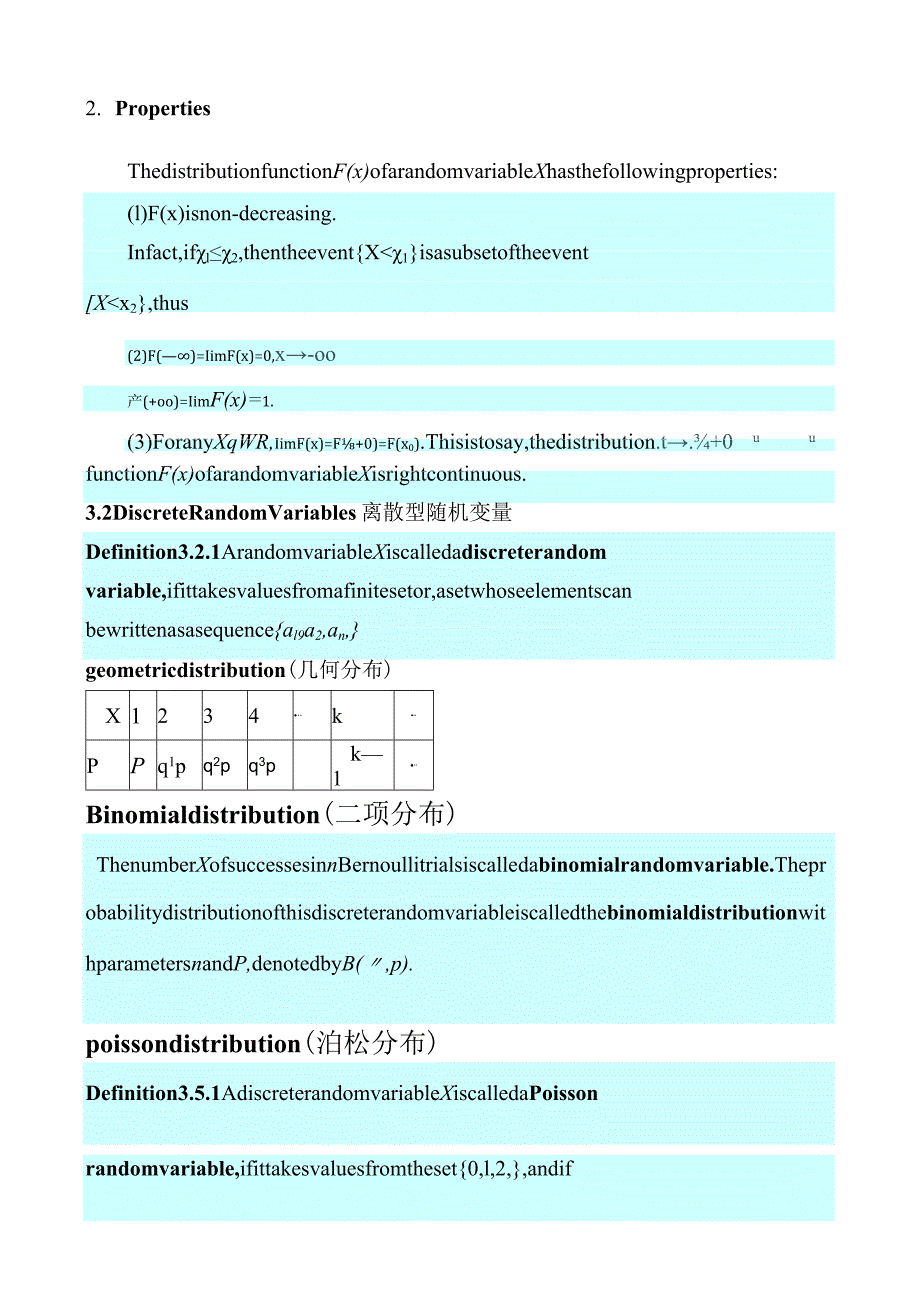
1、SampleSpace样本空间Thesetofallpossibleoutcomesofastatisticalexperimentiscalledthesamplespace.Event事件Aneventisasubsetofasamplespace.certainevent(必然事件)：ThesamplespaceSitself,iscertainlyanevent,whichiscalledacertainevent,meansthatitalwaysoccursintheexperiment.impossibleevent(不可能事件)：Theemptyset,denotedby0,i
2、salsoanevent,calledanimpossibleevent,meansthatitneveroccursintheexperiment.Probabilityofevents(概率)Ifthenumberofsuccessesinntrailsisdenotedbys,andifthesequenceofrelativefrequenciess/nobtainedforlargerandlargervalueofnapproachesalimit,thenthislimitisdefinedastheprobabilityofsuccessinasingletrial.equal
3、lylikelytooccur”probability(古典概率)IfasamplespaceSconsistsofNsamplepoints,eachisequallylikelytooccur.AssumethattheeventAconsistsofnsamplepoints,thentheprobabilityPthatAoccursisMutuallyexclusive(互斥事件)Definition2.4.1EventsA,A2,Aarecalledmutuallyexclusive,ifAiAj=0,Xfij.Theorem2.4.1IfAandBaremutuallyexclu
4、sive,thenP(AB)=P（八）+P(B)(2.4.1)Mutuallyindependent事件的独立性TwoeventsAandBaresaidtobeindependentifOrTwoeventsAandBareindependentifandonlyifP(BA)=P(B).ConditionalProbability条件概率Theprobabilityofaneventisfrequentlyinfluencedbyotherevents.DefinitionTheconditionalprobabilityofB,givenA,denotedbyP(3A),isdefine
5、dbyP(BlA)=P(；(AFifP（八）0.(2.5.1)Themultiplicationtheorem乘法定理If142,Aareevents,thenIftheeventsl42,4kareindependent,thenforanysubsetzl2,1,2,(全概率公式totalprobability)Theorem2.6.1.IftheeventsB1,B2,纥constituteapartitionofthesamplespaceSsuchthatP(Bj)0forj=1,2,k,thanforanyeventAOfS,kkP（八）=2P(ABj)=P(Bj)P(ABj)(2
6、.6.2)j=lj=(贝叶斯公式Bayes,formula.)IftheeventsB1,B2,BkconstituteapartitionofthesamplespaceSsuchthatP(Bj)Ofor)=1,2,k,thanforanyeventAofS,P（八）O,P(IlA)=/(耳)P(AIBLfOrf=1,2,yk(2.6.2)P(Bj)P(AIJ)j=ProofBythedefinitionofconditionalprobability,Usingthetheoremoftotalprobability,Wehave1. randomvariabledefinitionAr
7、andomvariableisarealvaluedfunctiondefinedonasamplespace;1 .e.itassignsarealnumbertoeachsamplepointinthesamplespace.Distributionfunction1.etXbearandomvariableonthesamplespaceS.ThenthefunctionF(X)=P(Xx).xeRiscalledthedistributionfunctionofXNoteThedistributionfunctionF(X)isdefinedonrealnumbers,notonsam
8、plespace.2. PropertiesThedistributionfunctionF(x)ofarandomvariableXhasthefollowingproperties:(l)F(x)isnon-decreasing.Infact,ifl2,thentheeventX1isasubsetoftheeventXOZ=O,1,2,k1) .5.1)Distribution(3.5.1)iscalledthePoissondistributionwithparameter,denotedbyP().Expectation(mean)数学期望Definition3.3.1LetXbea
9、discreterandomvariable.TheexpectationormeanofXisdefinedas=E(X)=ZxP(X=JV)(3.3.1)X2) Variancestandarddeviation(标准差)Definition3.3.2LetXbeadiscreterandomvariable,havingexpectationE(X)=.ThenthevarianceofX,denotebyD(X)isdefinedastheexpectationoftherandomvariable(X-/)2O(X)=E(X-M2)(3.3.6)Thesquarerootofthev
10、arianceD(X),denotebyJo(X),iscalledthestandarddeviationofX:yD(X)=(eX-)2V(3.3.7)probabilitydensityfunction概率密度函数Afunctiondefinedon(-oo,)iscalledaprobabilitydensityfunctionIS率密度函数)if:(i)f(x)OforanyxeR；OO(h)fi,x)isintergrable(可积的)on(-,)andJf(x)dx=1.-CODefinition4.1.21.et(x)beaprobabilitydensityfunction.IfXisarandomvariablehavingdistribulionfunctionF(x)=P(Xx)=ftdt,(4.1.1)-00thenXiscalledacontinuousrandomvariablehavingdensityfunctionX).Inthiscase,P(x1X0),ifitsdensityfunctionisgivenby1W、eforx0fM=(4.5.1)0forx0ThemeanandvarianceofacontinuousrandomvariableXhavingexponentialdistrib
